Read and Record Measurements With the Proper Sig Figs
 | Math Skills Review Meaning Figures |
In that location are ii kinds of numbers in the world:
- exact:
- instance: There are exactly 12 eggs in a dozen.
- example: Most people take exactly 10 fingers and 10 toes.
- inexact numbers:
- instance: whatever measurement.
If I quickly mensurate the width of a piece of notebook paper, I might become 220 mm (2 meaning figures). If I am more precise, I might get 216 mm (3 significant figures). An even more precise measurement would exist 215.six mm (4 significant figures).
- instance: whatever measurement.
PRECISION VERSUS Accurateness
Accuracy refers to how closely a measured value agrees with the correct value.
Precision refers to how closely individual measurements agree with each other.
In whatsoever measurement, the number of pregnant figures is critical. The number of significant figures is the number of digits believed to be right by the person doing the measuring. It includes i estimated digit. And then, does the concept of pregnant figures deal with precision or accurateness? I'll reply this question later you peruse the next example.
Allow's await at an example where pregnant figures is of import: measuring volume in the laboratory. This can be done in many ways: using
- a beaker with volumes marked on the side,
- a graduated cylinder, or
- a buret.
A dominion of thumb: read the book to 1/10 or 0.one of the smallest partition. (This rule applies to any measurement.) This means that the error in reading (called the reading error) is 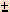 1/ten or 0.1 of the smallest division on the glassware. If you lot are less sure of yourself, you can read to 1/5 or 0.2 of the smallest division.
1/ten or 0.1 of the smallest division on the glassware. If you lot are less sure of yourself, you can read to 1/5 or 0.2 of the smallest division.
| Beaker | 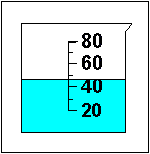 | The smallest division is x mL, so we can read the volume to 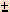 1/10 of ten mL or 1/10 of ten mL or 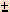 i mL. The volume we read from the beaker has a reading fault of i mL. The volume we read from the beaker has a reading fault of 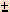 1 mL. 1 mL.The volume in this beaker is 47 So, How many significant figures does our volume of 47 |
| Graduated Cylinder |  Look in the textbook for a motion-picture show of a graduated cylinder. | First, note that the surface of the liquid is curved. This is called the meniscus. This phenomenon is acquired by the fact that h2o molecules are more attracted to drinking glass than to each other (adhesive forces are stronger than cohesive forces). When we read the volume, we read it at the BOTTOM of the meniscus. The smallest segmentation of this graduated cylinder is one mL. Therefore, our reading mistake will exist How many pregnant figures does our answer take? 3! The "3" and the "6" we know for certain and the "5" nosotros had to approximate a fiddling. |
| Buret | 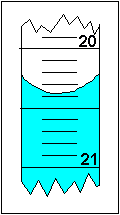 Look in the textbook for a picture of a buret. Note that the numbers get bigger every bit you lot become down the buret. This is unlike from the beaker or the graduated cylinder. This is because the liquid leaves the buret at the bottom. | The smallest sectionalization in this buret is 0.i mL. Therefore, our reading fault is 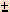 0.01 mL. A good volume reading is 20.38 0.01 mL. A good volume reading is 20.38 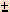 0.01 mL. An as precise answer would be 20.39 mL or twenty.37 mL. 0.01 mL. An as precise answer would be 20.39 mL or twenty.37 mL. How many significant figures does our answer have? 4! The "two", "0", and "3" we definitely know and the "eight" we had to approximate. |
Conclusion: The number of meaning figures is straight linked to a measurement. If a person needed only a rough estimate of volume, the beaker volume is satisfactory (2 meaning figures), otherwise one should use the graduated cylinder (3 significant figures) or better yet, the buret (four significant figures).
Then, does the concept of significant figures bargain with precision or accuracy? Hopefully, you tin can see that it actually deals with precision simply. Consider measuring the length of a metal rod several times with a ruler. Yous will go essentially the same measurement over and over over again with a small reading mistake equal to about 1/ten of the smallest division on the ruler. Y'all have determined the length with high precision. Withal, you don't know if the ruler was accurate to begin with. Perhaps it was a plastic ruler left in the hot Texas sun and was stretched. You lot don't know the accuracy of your measuring device unless y'all calibrate information technology, i.e. compare information technology against a ruler you lot knew was accurate. Note: in the laboratory, a good analytical chemist always calibrates her volumetric glassware before using it by weighing a known book of liquid dispensed from the glassware. By dividing the mass of the liquid by its density, she tin can make up one's mind the actual volume and hence the accuracy of the glassware.
Rules for Working with Significant Figures:
- Leading zeros are never significant.
Imbedded zeros are e'er pregnant.
Trailing zeros are meaning only if the decimal indicate is specified.
Hint: Change the number to scientific notation. It is easier to run across. - Addition or Subtraction:
The last digit retained is set up by the first doubtful digit. - Multiplication or Division:
The answer contains no more significant figures than the to the lowest degree accurately known number.
EXAMPLES:
| Instance | Number of Significant Figures | Scientific Annotation | ||
| 0.00682 | 3 | 6.82 x x -iii | Leading zeros are not meaning. | |
| 1.072 | four | i.072 (ten 100) | Imbedded zeros are always significant. | |
| 300 | ane | 3 x tentwo | Trailing zeros are significant only if the decimal point is specified. | |
| 300. | three | 3.00 x 10ii | ||
| 300.0 | 4 | 3.000 x 102 |
EXAMPLES
| Add-on |  | Even though your reckoner gives you the respond 8.0372, yous must round off to 8.04. Your answer must only contain 1 doubtful number. Notation that the doubtful digits are underlined. |
| Subtraction |  | Subtraction is interesting when concerned with significant figures. Even though both numbers involved in the subtraction have five significant figures, the answer simply has iii pregnant figures when rounded correctly. Call up, the reply must simply have 1 doubtful digit. |
| Multiplication |  | The reply must be rounded off to 2 significant figures, since 1.6 but has 2 significant figures. |
| Division |  | The answer must exist rounded off to 3 meaning figures, since 45.ii has only 3 pregnant figures. |
Notes on Rounding
- When rounding off numbers to a certain number of significant figures, do so to the nearest value.
- example: Circular to 3 meaning figures: 2.3467 x 104 (Answer: 2.35 10 104)
- example: Circular to 2 significant figures: i.612 x 10iii (Answer: 1.6 10 10three)
- What happens if in that location is a five? At that place is an arbitrary rule:
- If the number earlier the v is odd, round up.
- If the number before the five is even, let it be.
The justification for this is that in the form of a serial of many calculations, any rounding errors will be averaged out. - example: Round to ii significant figures: 2.35 x ten2 (Answer: ii.four x x2)
- example: Circular to 2 significant figures: 2.45 x ten2 (Answer: 2.four x ten2)
- Of grade, if we round to 2 significant figures: two.451 10 x2, the answer is definitely ii.5 x 102 since two.451 x 10ii is closer to 2.five x 102 than 2.4 x x2.
QUIZ:
| Question one | Give the correct number of significant figures for 4500, 4500., 0.0032, 0.04050 |
| Question ii | Requite the answer to the correct number of pregnant figures: 4503 + 34.xc + 550 = ? |
| Question 3 | Give the respond to the correct number of significant figures: ane.367 - one.34 = ? |
| Question 4 | Give the answer to the correct number of significant figures: (1.3 x 10three)(v.724 x 10iv) = ? |
| Question 5 | Give the reply to the right number of significant figures: (6305)/(0.010) = ? |
Answers: (1) two, four, 2, 4 (2) 5090 (iii significant figures - round to the tens place - fix by 550) (3) 0.03 (1 significant effigy - round to hundredths place) (4) 7.4 ten 10vii (2 meaning figures - set by i.3 x 103) (5) 6.3 x x5 (two significant figures - set past 0.010)
Pick your next topic:
granberrythaniorefore1998.blogspot.com
Source: https://www.chem.tamu.edu/class/fyp/mathrev/mr-sigfg.html
Posting Komentar untuk "Read and Record Measurements With the Proper Sig Figs"